Lūkesčiai, Palyginimai, Dievo įsivaizdavimas, Gyvybės dėsniai, Vertybių žemėlapis, Kūrybos prasmė ir meno taisyklės, Dvylika klausimų
Kaip užtikrintam Dievui išgyvenant lūkesčius apibrėžti aplinkybes?
Nusižiūrėjus į tiesos atvejį (akivaizdumas = turinio ir raiškos atitikimas) palyginti kaip kiti nulybės atvaizdai suveikia (ar pasidalina) aplinkybes apibrėžiančiuose žaidimuose.
Glaudžiau susieti aplinkybes su trejybės ratu.
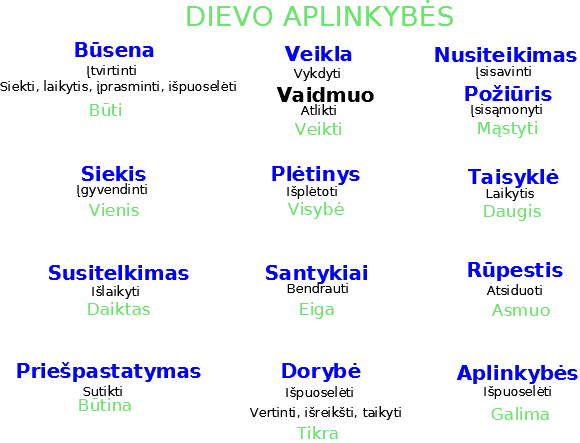
Suprasti pavyzdžius dvylika aplinkybių.
- Kaip Dievą įsivaizduojame psalmėse.
- Lakoff ir Johnson palyginimuose.
- MenoTikslas
- MenoAplinkybės
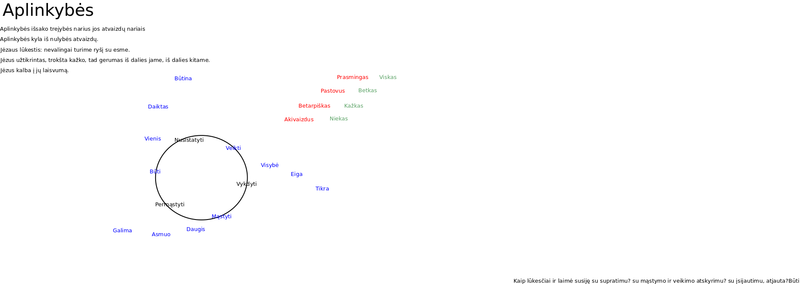
Kur yra tiesa, betarpiškumas, pastovumas, prasmingumas?
- Tiesa nusistatyme (būtinumas), vykdyme (tikrumas), permąstyme (galimumas).
- Betarpiškumas nusistatyme (daiktas), vykdyme (eiga), permąstyme (asmuo).
- Pastovumas nusistatyme (vienis), vykdyme (visybė), permąstyme (daugis).
- Prasmingumas nusistatyme (buvimas), vykdyme (veikimas), permąstyme (mąstymas).
Užrašai
- Galimybė yra neigimo neigimas - nėra taip, kad nėra tiesa.
- Teiginių pagrindas yra tiesos žaidimas, juk eina kalba apie teiginio tiesą.
Aplinkybių pavyzdžiai:
- trejybės atvaizdai
- gyvybės savybės
- 12 klausimų
- psalmėse
- palyginimuose (Lakoff ir Johnson)
- Dievo aplinkybėse - maldose
- veiksmažodžių priešdėliuose
- 4 geometrijos - trikampiai
Aplinkybėmis vadinu dvylika topologijų, tai ką Kantas vadino kategorijomis. Žr. Vikipedijos straipsnį. Kategorijos (predikatai) yra tai, ką galima apie betką pasakyti. Aš jas vadinu topologijomis ar aplinkybėmis, pabrėždamas, jog tai ne daiktų apibendrinimai, o verčiau jų fonai, kuriais juos įsivaizduojame. Tai vaizduotės žodynas, sąvokynas.
Aplinkybės taip pat yra sąlygos (circumstances).
Aplinkybės yra atsakymai į klausimus:
- Kodėl? Kodėl!: esu, veikiu, mąstau
- Kaip? Kaip!: vienis, visybė, daugis
- Koks? Koks!: daiktas, eiga, asmuo
- Ar? Ar!: būtinas, tikras, galimas
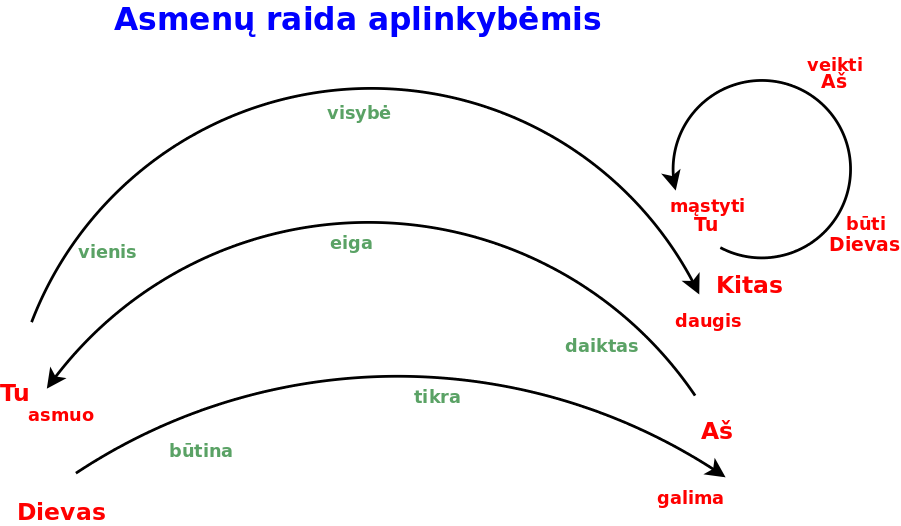
Viskas apima keturis lygmenis ir jie jį padalina. Nulybės atvaizdai išreiškia nulybę ir šių keturių atvaizdų vieningumas išreiškia meilę.
- Akivaizdumas neigia Ar ir atvirkščiai
- Betarpiškumas neigia Koks ir atvirkščiai
- Pastovumas neigia Kaip ir atvirkščiai
- Prasmingumas neigia Kodėl ir atvirkščiai
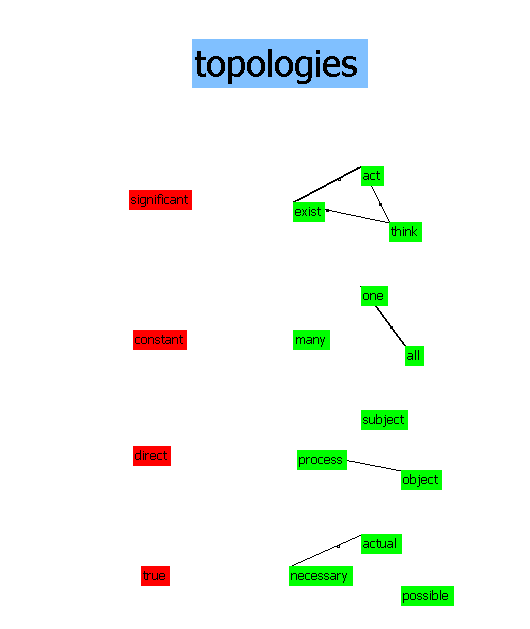
There are twelve topologies. They are the vocabulary of our imagination. They are the perspectives given by the four RepresentationsOfTheThreesome, generated by the four RepresentationsOfTheNullsome, as follows:
- exist, act, think generated from significant
- one, all, many generated from constant
- object, process, subject generated from direct
- necessary, actual, possible generated from true
Note that significant (not encompassed), constant (not changing), direct (not mediated), true (not hidden) are negations of the four perspectives of the {{Foursome}}. They are also the four RepresentationsOfTheNullsome and the PropertiesOfGod.
We may think of {{Everything}} and {{Anything}} as players that are trying to connect. As anything, we are limited, hence unable to conceive directly our connection, but may only allude to it. Our conception is thus given not by this infinite recursion, but through representations of everything. Everything is represented in four ways, as true, direct, constant, significant. Moreover, in its relationship with anything, it is given as a player of a mind game which generates properties of life, of the oneness of the spirit beyond and within structure, of everything and anything.
"Significant" generates a mind game, where significant is that which is unencompassable, can't be captured: If thinking is significant, then so is being, for thoughts are encompassed by the thinker. If being is significant, then so is doing, for things are encompassed by the actions that manifest them. If doing is significant, then so is thinking, for actions are encompassed by the observations made of them.
"Constant" generates a mind game: In searching for constancy, we find one example of constancy, otherwise it is constantly unconstant. So the constancy is in the "one" or in the "all". Note that to do this search, what we select needs to be the same as what we judge, they are multiply constant, "many".
"Direct" generates a mind game: Our attention can be directed by something other than itself, an "object". Subsequently, it can be directed by itself, this is "process". Finally, it may or may not be directed, this is "subject".
"True" generates a mind game, where true is what can't be hidden, is obvious: The content and what reveals it may be the same, in which case it is "necessarily" true. Otherwise, the content may be different from what reveals it, and follows it, so is "actually" true. Finally, what may be true is the relationship between the content and what reveals it, which is "possibly" true.
Aristotelio tiesa: akivaizdumas = raiškos ir turinio atitikimas (o tai iškyla žaidimu).
3 trejybės atvaizdai yra hegeliniai (Hegelio: tezė, antitezė, sintezė) ir 1 yra dekartinis (Dekarto: mąstau, vadinas esu; esu, vadinas veikiu; veikiu, vadinas mąstau). Hegeliniai pagrįsti neigimu (vienis pastovus, visybė pastoviai nepastovus; daiktas, tai dėmesys kitam, o eiga, tai dėmesys sau pačiam; būtina kada sutampa turinys ir raiška, tikra kada nesutampa). Dekartiniai nepagrįsti neigimu.
Self-Consciousness in Kant's Transcendental Deduction of the Categories
- Kit Slover at St John's College, Dean's Lecture & Concert Series, 2021.
- https://plato.stanford.edu/entries/kant-transcendental/
- https://www.jstor.org/stable/2249498 Ewing
- https://philpeople.org/profiles/kit-slover
- Time and Transcendental Matter: The Sensible Role of the Thing in Itself
Kantas taikė kategorijas sakinių - teiginių turiniui - keturiuose lygmenyse. Mąstome sakiniais.
- logical functions of judgment tarnauja kaip gamtos kategorijos - transcendental deduction
Platesnis židinys pasitraukia, kad siauresnis galėtų iškilti. Pasitraukimas yra prasmingumo paneigimas. Ieškojimas yra pastovumo paneigimas. Dėmesys (tarpas) yra betarpiškumo paneigimas.
Lyginu laipsnynus? su aplinkybėmis.
Įrodymų būdus sieju su aplinkybėmis vienis-daugis-visybė. Jos išplaukia iš pastovumo. O jie yra įvairūs būdai kaip suprantame funkciją, kaip ja pristatoma Dievas už santvarkos ir gerumas santvarkoje.
- Funkcija priskiria reikšmę vienai paskirai reikšmei. Priskyrimas kuria santvarką morfizmu ir vyksta santvarkoje substitucija.
- Funkcija daugeliui įvesčių priskiria reikšmes lygiagrečiai. Priskyrimas kuria santvarką indukcija ir vyksta santvarkoje atvejų nagrinėjimu.
- Funkcija įvesčių visumai priskiria savo būdą, savo esybę. Priskyrimas kuria santvarką algoritmo konstravimu ir vyksta santvarkoje konstravimu.
Būti, veikti, mąstyti yra ciklinė trejybė nes atitinkama apimtis yra viskas. Užtat kiti atvaizdai nesudaro rato nes atitinkamos apimtys yra siauresnės.
Kantas tvirtino, kad nėra aposteriori analitinių teiginių, tai yra, sandarioje santvarkoje nėra ką pažinti. Gal dėl to jisai ir nepripažino Dekarto "mąstau, vadinas esu". Betgi savo kailiu patyriau, kad jeigu mąstymas neaprėpiamas, tai ir buvimas neaprėpiamas. Tai yra, jei mintys neaprėpiamos, tai mąstytojas juo labiau. O jeigu buvimas neaprėpiamas, tai ir veikimas neaprėpiamas. Juk tėra tai, kas reiškiasi. Ir jei veikimas neaprėpiamas, tai ir mąstymas neaprėpiamas, nes visus veiksmus galima apmąstyti. Šį trejybės ratą - buvimo, veikimo, mąstymo - pažįstame iš gyvenimo. Jisai išplaukia iš neaprėpiamumo, kurį išgyvename, kaip prasmingumą: jei mąstymas prasmingas, tai ir buvimas prasmingas, ir t.t. Neaprėpiamumą, požiūrio nepakankamumą, įsivaizduoja tik tas, kuris santvarkos varžomas, joje gyvena, patiria jos ribas, nesutelpa jose ir ieško sąlyčio su tuo, kas už jų.
- GodTheFather is the Necessity of God
- GodTheSon is the Actuality of God
- GodTheSpirit is the Possibility of God
Aplinkybių kildinimas
{{Andrius}}: The historical notes further below for an explanation of how I came to the topologies above. In this section, I share my work to derive the topologies from the {{Omniscope}}.
The topologies are what relate {{Anything}} and Something. We may think of anything as an {{Observer}}, and something as an {{Observed}}. Something is in its own ObservationalPlane. But now consider it in a larger plane, the one that is observed by {{Anything}}. The topologies express the various ways that anything and something may thereby be related.
The topologies are one of the six SecondaryStructures. Each of the secondary structures arises from God of a more accessible PrimaryStructure interpreting a less accessible primary structure. In this way, God sees something more than simply himself, so that his view coincides with the observer for that less accessible primary structure.
The topologies are the secondary structure where God who has access to {{Anything}} (and is certain) interprets the primary structure which provides access to {{Something}} (and describes our {{Expectations}}). In this sense, we imagine (and thus coincide with the view) that God is anything and he is taking up the ways of pulling away from something.
The ways of pulling away from something are given by the primary structure [{{Everything}} wishes for anything]. They are given by the six {{Expectations}}, which are the DirectionsFromTheGood:
- good God
- good quality
- good gift
- good person
- good deed
- good word
Here they are understood as expressing good will, an other beyond us. We are expecting the good, and these are the directions from which the good might come.
If these directions from the good are interpreted by God who has access to everything, then such a God wishes for nothing. Hence, all of the goodness is then not in God, but rather in the one who opens themselves up to God. The directions from the good then are interpreted as the {{Representations}}.
However, if instead they are interpreted by God who has access to anything, then such a God wishes for something. Then the good is both in us and in God. It is the good of God (the anything in one of four planes) as it is isolated within that plane as one of three modes (yielding a topology). Thus it is the good of the good, as it has been isolated. This is related to our speaking to the good in others, and the DirectionsToTheGood become relevant.
The topologies are derived from the empathy that "Everything that wishes for something" has for "the not-wishing of anything". The latter is given by the directions that the good may come from, as these are the expectations in not wishing for just anything. What does everything wish for? That anything indeliberately be connected with the essence, so that all connects back unto everything. This is also that people follow the will of God. So this is a coinciding of the will of God, and the will of a person. Inasmuch as everything wishes for something, there is a domain for that wish, and therefore a representation of everything, which is given by everything, one of four representations, which are wishes. And as anything may follow the will of everything, there are three reasons for doing so: obeying, believing, caring. These together make for the twelve topologies.
Topologies are derived when EverythingWishesForAnything.
Look for two secondary structures which come from this: Criteria and Topologies.
They involve "waiting" and "believing" as their seventh perspectives.
Good person, deed, word are a cycle:
- Good person: reflect => take a stand
- Good deed: take a stand => follow through
- Good word: follow through => reflect
Good God, gift, quality get mapped to follow through, reflect, take a stand.
- Good God is what lets the good person do the good deed, they are cocreators.
- Good gift is what lets the good word judge the worker by the work, judge the good deed.
- Good quality is what distinguishes the good person on the basis of the good word, throws away the bad and preserves the good.
In those roles, good God is one, good gift is all, good quality is many.
Representation has us have expectations, God wish for nothing, and has "waiting" as its seventh perspective. If God wishes for nothing, then look for good in people. Everything is self-sufficient. Waiting = being in suspense, as an emotional response.
Topologies have us have expectations, God wish for something, and have "believing" as its seventh perspective. If God wishes for something (connection), then look for good in both God and people. Everything is certain. Believing = sheer will, for getting things done.
Good will rests in two locations. We address the good will in others, and we show good will ourselves.
The directions of the good are the structure for the expectations.
In each case, the slack is in the direction of the good:
- good heart = Jesus' belief, rather than his wish (goodness of nullsome)
- good God = Jesus' willingness to change, rather than unwillingness to change (goodness of onesome)
- good gift = Jesus' investedness, rather than his interest (goodness of twosome)
- good quality = Jesus' isolating, rather than his isolated (goodness of threesome)
- good person = Jesus' inner adherence, rather than his outer adherence (goodness of foursome)
- good deed = what Jesus will achieve, rather than what he is able to do (goodness of fivesome)
- good word = what Jesus could be doing, rather than what he is doing (goodness of sixsome)
- good news = Jesus' purpose, rather than his strength (goodness of sevensome)
Slack is in the former, not the latter. Slack is in the qualities of signs:
- malleable = slack is in their willingness to change
- modifiable = slack is in their inertia
- mobile = slack is in their isolator
- memorable = slack is in their inner adherence
- meaningful = slack is in what they will achieve
- motivated = slack is in what they could be doing
Expectations are given by the gradation of methods of mathematical proof: morphism, induction, algorithm construction, substitution, examination of cases, construction. These seem to define the various kinds of goodness:
- morphism is the goodness of God
- induction is the goodness of gift
- algorithm construction is the goodness of quality
- substitution is the goodness of person
- examination of cases is the goodness of deed
- construction is the goodness of word
The good will that we show is actually that which we open through the eight directions which the good comes from. These are the same directions that we speak to in others.
We can speak to the good will in others. Or we can open the way for it to speak from us. For example, we can go from "unwillingness to change" to "willingness to change", or the reverse.
Each of the directions of the good accords as if with a division of everything. Depending on the direction that we look at them, we have either representations or topologies. What do we associate the good with? the slack with? in a division. Either with the part, or with the whole. If with the part, then that part stands independently, and we have a topology. If with the whole, then the whole stands independently, and we have a representation. Whatever we associate the good with must stand independently on either side.
Representations and Topologies
A division relates a whole with its parts.
The good is related to either the whole or the part.
Jesus is Good will.
Good will is in the whole: representations.
Good will is in the part: topologies.
Criteria have to do with increasing slack. Criteria: A perspective (ours - the part) sees The perspective (God's - the whole). We open the way for the good, and it keeps pouring out.
Representations are the good will that we show. Good is in the whole.
Opening to the ways of the good is given by the emotional responses.
Criteria (representations) arise here when God wishes for nothing. This is the goodness of the whole, of the representation. It opens up the way for goodness. Good quality is the good of decreasing slack, good word is the good of increasing slack. Good God is the good of why, good person is the good of how, good deed is the good of what, good gift is the good of whether.
Topologies are the good will that we speak to. Good is in the part.
Topologies arise here when God wishes for something. This is the goodness of the part, of the topology. It speaks to the goodness within the person. I need to think about this!
Speaking to the good is done by the ways of getting things done.
The key concepts significant, constant, direct, true are given by the good that Jesus speaks to:
- significant = their willingness to change (not take a stand)
- constant = their inertia (not follow through)
- direct = their isolation (not reflect)
- constant = their inner adherence (take a stand)
- direct = what they will achieve (follow through)
- true = what they could be doing (reflect)
The threesome is given by: good God, gift, quality or good person, deed, word. Question: Where is the good x in the good y? Also, how might these relate to the topological extensions?
- Good God (significant) => Good person, deed, word: be do think
- Good Gift (constant) => Good deed, word, person: one all many
- Good Quality (direct) => Good word, person, deed: object process subject
- Good Person (constant) => Good God, gift, quality: one all many
- Good Deed (direct) => Good gift, quality, God: object process subject
- Good Word (true) => Good quality, God, gift: necessary, actual, possible
[[table] good |Jesus' |nullsome |good |topology |good |topology |good |topology God |willingness to change |significant |person |be |deed |do |word |think gift |inertia |constant |person |one |deed |all |word |many quality |isolator |direct |person |object |deed |process |word |subject person |inner adherence |constant |God |one |gift |all |quality |many deed |what they will achieve |direct |God |object |gift |process |quality |subject word |what they could be doing |true |God |necessary |gift |actual |quality |possible ]
Here the topologies are narrowing down where exactly is the good. They are narrowing it down to a particular part, one of three. In this way, they isolate that particular part, allow us to consider it distinct from the others. Also, to do this, its good must be with regard to a particular plane, one of four, that it is attributing the good to. Hence the good is both in the plane and in the part, whence it is indeed slack, good. And that plane must be considered from either God's or a person's point of view, as possible.
- For the good God: the good person is be, the good deed is do, and the good word is think.
- For the good gift: the good person is one, the good deed is all, and the good word is many.
- For the good quality: the good person is the object, the good deed is the process, and the good word is the subject.
- For the good person: the good God is one, the good gift is all, and the good quality is many.
- For the good deed: the good God is object, the good gift is process, and the good quality is subject.
- For the good word: the good God is necessary, the good gift is actual, and the good quality is possible.
This uses the directions of the good as a framework to pair a representation of the nullsome with a threesome, attributing good to the perspectives, yielding the three topologies. We have the threesomes as they look from a perspective on the other side.
A topology is gotten by representing the entire threesome, and taking one of the perspectives
Topologies have to do with decreasing slack, with reducing good to zero. This leaves structure, the part. Topologies: The perspective (God's - the whole) see A perspective (ours - the part).
With topologies: The whole (whatever is the direction of the good) gets mapped to the representation of the nullsome, and accordingly, the part gets mapped to a topology. Here good gets reduced to zero.
Perhaps more precisely, for example: (the perspective) good God (the direction of the good) gets understood as good + whole, and when the good gets sent to null, we have the whole become a representation of the nullsome. And then accordingly, (a perspective) for good person, deed, word, we have that it's understood as good + part, and as the good goes away, we are left with a topology, and they can be understood collectively as a representation of the threesome.
Jesus' willingness to change => their willingness to change = significant
Now I need to work out specifically the various topologies to make sure this approach makes sense.
Note the connection between good person/deed/word = = inner adherence/what they will achieve/what they could be doing = = take a stand/follow through/reflect. (or is it just be do think ?) Consider also if there is a connection between: good God/gift/quality = = willingness to change/investedness/isolation = = follow through => reflect / take a stand => follow through / reflect => take a stand.
Study how Jesus addresses, and shows, good will.
Note that, for example, Jesus' wish speaks to their belief, and then the Good heart speaks to their wish. This suggest that the Good heart is Jesus' belief, and so on. Also, note that this is the "belief" that we are looking for, as the basis for the topologies.
===Ideas for Deriving the Topologies===
Note that here God is certain.
Consider the good of the good as relating the good of anything and the good of something.
===Historical Notes===
{{Andrius}}: I collected many examples of each of the DivisionsOfEverything, especially the {{Twosome}}, {{Threesome}} and {{Foursome}}. I noticed that, conceptually, the examples seemed to fall into groupings. I started to think in terms of {{Representations}} of the divisions. At this time, in 1988, Rolandas Pavilionis introduced me to Immanuel Kant's Critique of Pure Reason. I noticed that his twelve categories were quite similar to the set of four RepresentationsOfTheThreesome which I had been observing. Gradually, I concluded that these twelve had significance in their own right, and as such would, along with the eight divisions and six representations, yield a comprehensive system based on portions of 2 x 3 x 4 = 24, along with three languages that shifted between them. I thought that the twelve categories were what allowed us to distinctly conceive a single part of a division, so that we might think of it separately from the others, just as the representations allow us to distinctly conceive the whole.
In 1993-1994 I worked to account for the twelve (and not necessarily Kant's twelve). I had concluded that Kant's argument in the Critique of Pure Reason was incoherent, or simply missing, at a few key places in his Transcendental Deduction, and in some important matters just plain wrong (especially where he argues that there are no analytic a posteriori statements; I think that be, do, think (and associated Cartesian statements such as if thinking is unencompassable, then being is unencompassable, if being is unencompassable, then doing is unencompassable, if doing is unencompassable, then thinking is unencompassable) are analytic (a closed system) and a posteriori (understood by way of experience). I surveyed readings in metaphor, rhetoric and emotion. I also did an empirical study of the first forty psalms, noting and sorting all of the ways that God is conceived, with the thought that God is as undefined as any universal dummy variable might be, and so our imaginations of him simply reflect the limits on our own imaginations, which are reached by the intensity of the psalms. I was very much helped by Lakoff and Turner's thoughts on metaphor, in that I came to think that the whole idea of category was misconceived, inasmuch as it is an abstract thing that we imagine. A key problem (as I learned from [{{TopologiesInPsalms}} grouping my data from the psalms]) was that there was generally not a single word or sentence that could summarize the abstraction. And there might not be any particular set of rules that was canonical. Instead, there was a definite world, a canvas, a backdrop, a mental space that could be defined. At this point, I started to call them topologies, as they are, so to speak, rules for such worlds. It is as if we may imagine what it is like to live on a sphere, or a plane, or a torus, and so on. Our imagination projects such topologies as backdrops for whatever we choose to imagine, which may be nothing at all. In this way, they are the vocabulary for our imagination. Next, after deep thinking, I realized that the topologies in the triplet (one, all, many) could be derived with regard to each other, as the outcomes of a mind game: the search for constancy. I saw that constancy was a trigger that yielded such a mind game (search for constancy - either you find one example of it (and the constancy is in that one), or you don't find any example (and so it is constantly inconstant, and the constancy is in the whole), and furthermore, in order to play this game, whenever you select an example to inspect, and then conclude upon inspection, you presume that the two are the same, and that this is generally the case (hence multiply constant). (This particular mind game is often applied in the theory of recursive functions and the study of Turing machines). Finally, I noticed that constant means not changing, hence negates the level of the {{Foursome}} that is how and is interpreted as creation, causation, utility, and such. So I was able to negate the other three levels and work from the resulting triggers to look for analogous mind games that they might yield. In particular, this lead to a definition of true as what is not hidden, what is obvious, that for which there is no whether. I worked out four mind games, and was happy that the four triggers were associated to the levels of the {{Foursome}} and thus clarified why there were exactly twelve topologies, and what they were. It became apparent that taking a stand, following through, and reflecting were not topologies but rather the three perspectives that made up the {{Threesome}} and which were then represented by the four RepresentationsOfTheThreesome, each of which was a mind game. Also, I noted that the four triggers made sense as the four representations of the {{Nullsome}} and were thus distinct from the four representations of the {{Onesome}}. This clarified the distinction between the PropertiesOfGod and the PropertiesOfEverything. I also did an empirical study of all of the metaphors in Metaphors We Live By, grouping them into [{{TopologiesInMetaphors}} twelve metaphorical ideas].
Later I made progress in realizing that there was not one but four PrimaryStructures and that the DivisionsOfEverything could be intepreted, and presumably derived, as injections of God from one level into the primary structure of another. I then worked to understand that primary structure EverythingWishesForAnything which yields the representations and the topologies. I did an empirical study of Jesus' emotional responses in the Gospel of Mark. I was able to relate them to DirectionsFromTheGood and DirectionsToTheGood. In 2004, I was able to express the representations and the topologies in such terms in a plausible way. After working on the {{Omniscope}}, I now return to consider precisely how the topologies arise. All of this work prepares the way for understanding the three {{Languages}}.
Aplinkybės yra dviprasmiškos. Jas galima suvokti, kaip atsitokėjimus (kūrybos tikslus - kodėl) arba kaip įsijautimus (meno taisykles - kaip).
- Aplinkybės išplaukia iš nulybės atvaizdų, tad tai yra ryšys su Dievu (nulybe), o gal ir su gerumu (atvaizdu).
3 x 4
- Trejybė - išoriniai santykiai: 3 + 3
- Ketverybė - vidiniai santykiai: 4 + 6.
Pavyzdžiai
Christopher Alexander - 15 gyvybės dėsnių. Apie juos sužinojau 2003 metais. I interpreted them as the three perspectives of the Threesome plus the twelve topologies. This interpretation is proving very helpful to intuit how the topologies relate Anything and Something, which I now recognize is their purpose.
Aplinkybės palyginimuose - Lakoff ir Johnson
Aplinkybės psalmėse
Kūrybos prasmė ir meno taisyklės - kur Dievas
Meilės moksle - kur Dievas
Proto darbo laukai
Kanto kategorijos kažkiek panašios. Chris Jones straipsnis
Anthony Judge - dvylikanaris protas?. Taip pat galvojau apžvelgti apžvelgti Anthony Judge mąstymą. Jisai sakė, kad tai geriausiai apžvelgia šis jo priešingybių sąrašas.
- Diversity -- Unity
- Agreement -- Disagreement
- Organization -- Chaos
- Configuration -- Relationships
- Strategy -- Lifestyle
- Static -- Dynamic
- Conventional -- Radical
- Future -- Present
- Global -- Personal
- Information systems -- Communication
- Comprehension -- Incomprehension
- Facts -- Aesthetics
- Balance -- Imbalance
- Problems -- Potential
- Prielaida: Trejybės atvaizde "vienis, visybė, daugis" daugis yra prielaida. Kituose trejybės atvaizduose, kas yra prielaida?
Kažin kada šitą nubrėžiau. Įdomu palyginti su 12 klausimų.
Kas yra kategorija? (Tadas Snuviškis)
- intensijos (sąlygų savybės - vaizduotės žodynas - aplinkybės) ir ekstensijos (iš aibių - apibendrinimai - narystė - priklausymas kategorijai)
Aplinkybė yra požiūrio esmė - apibrėžimas.
Langacker aplinkybės - image schemes - "configurational concepts"
- Aplinkybės - pirmloginės.
Thu, 06 Mar 2008 05:29:09 UTC ThomasChepaitis: topology is a science about a place ("topos" in Greek) I studied some time the theory of knots, in connection with Lacan's psychotherapy. Do you use topology in that sence? Place can be closed, ant space isn't, which was proved by Moebius, as I understand it
Užrašai
- https://en.wikipedia.org/wiki/Charles_Sanders_Peirce#Modes_of_inference
- Necessary, actual, possible: Deduction proves that something must be; Induction shows that something actually is operative; Abduction merely suggests that something may be.
- Induction seeks facts to test a hypothesis; abduction seeks a hypothesis to account for facts.
- Abduction is the reduction of surprise (thus reformulating emotional expectations). "The surprising fact, C, is observed; But if A were true, C would be a matter of course, Hence, there is reason to suspect that A is true."
- Abduction only takes place reactive when we are mistaken.
- Proactive experimentation (Andrius) vs. Reactive experimentation (Peirce). "Guesses can be selected for trial strategically" - an example of deduction in the stage of hypothesis forming.
- In proactive experimentation, the experimenting occurs in the "abduction" of following through. In reactive experimentation, the design of the experiment is deduction, and the conducting of the experiment is induction.
- Proactive experimentation - vystome save (pasąmonę). Reactive experimentation - vystome savo žinojimą.
- Proactive experimentation - nėra savasties, tad nėra ribos. Išgyvename ramybę. Reactive experimentation - tyrimas vyksta už mūsų, tad išgyvename nuostabą, nustembame.
- Dėmesingumas - awareness - suvokimas - savęs stebėjimas - reikalingas taikant Christopher Alexander derinius.
Antanas Klimas, Prefixes and Suffixes in the Lithuanian Verbal System - palyginti dvylika priešdėlių su aplinkybėmis:
- ap-
- at-
- į-
- iš-
- nu-
- pa-
- par-
- per-
- pra-
- pri-
- su-
- už-
dar reikėtų pridėti:
- ne-
- te-
- be-
- tebe-
- "da"-
Aplinkybės
Naujausi pakeitimai
网站
Įvadas #E9F5FC
Klausimai #FFFFC0
Teiginiai #FFFFFF
Kitų mintys #EFCFE1
Dievas man #FFECC0
Iš ankščiau #CCFFCC
Mieli skaitytojai, visa mano kūryba ir kartu visi šie puslapiai yra visuomenės turtas, kuriuo visi kviečiami laisvai naudotis, dalintis, visaip perkurti. - Andrius
